GEOSTATIONARY ORBITS PART 5:
INCLINED-ORBIT SATELLITES

SATELLITE DRIFT

A satellite intended for radio communications among fixed earth stations must meet two criteria:

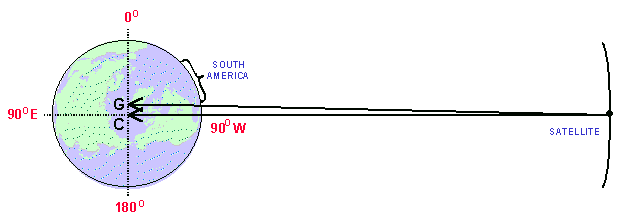A satellite at 90° west longitude.  Point C = geometric center.  Point G = apparent center of gravity.
Point G lies east of Point C because of the land mass of South America.  Note that the vector
to the earth's apparent center of gravity (Point G) is not congruent with the vector to
the earth's geometric center (Point C).  In the case of geostationary satellites visible from
North America, the land mass of South America tends to cause them to drift to the east.

ROCKETS

To counteract these forces, the satellite must be fitted with some mechanism to move the satellite back into position when it drifts. In outer space, only one such mechanism is available: the rocket.

Now, let's stop and discuss rockets. The popular conception of a rocket is something very large which burns some sort of fuel to produce an enormous thrust.

More generally, a rocket is any device which produces a force by ejecting mass. Fuel-burning rockets produce force by ejecting the products of combustion. But combustion isn't necessary: any mechanism for ejecting mass will produce a force. A common example: a loose balloon flying around the room ejecting its air supply.

The direction of the force produced by a rocket is equal and opposite to the force required to eject the mass. This, of course, is Newton's Third Law: for every force, there is an equal and opposite reaction force.

STATIONKEEPING

Communications satellites are fitted with small rockets called thrusters. On command from the a control station, a thruster is fired; during the firing, it ejects a gas called fuel or propellant. Like the air escaping from the balloon, the ejected gas produces the force.

A ground control station precisely controls all parameters involved in a firing: the position of each thruster relative to the satellite, the timing and duration of each fire, and the pressure of the ejected propellant. If these parameters are controlled properly, the satellite can be maintained at proper position and attitude for years.

This process is called stationkeeping.

SATELLITE LIFE EXPECTANCY

Every time a thruster is fired, propellant is used. Once the supply of propellant is exhausted, the satellite cannot be maintained at proper position and attitude, and the satellite must be retired. Propellant capacity is the primary factor which determines the useful life of a communications satellite.

It is easy to understand that a primary goal of every satellite owner is the conservation of propellant. Many computer studies have been done to determine the optimum trade-off between satellite stability and propellant usage.

These studies have shown that a substantial majority of the propellant is used for just one stationkeeping function: keeping the satellite from drifting along its north-south axis. Kent Carson, director of advanced programs for Comsat Systems Division, has stated that between 80% and 90% of the propellant is used for this function alone. [1]

INCLINED ORBITS

Let us now consider what happens if the owner of a satellite simply stops north-south stationkeeping, and lets the satellite drift freely along its north-south axis.

Two things happen.

• First, the orbit plane becomes inclined with respect to the earth's equatorial plane. The following figure illustrates this situation.

During the course of one sidereal day, the satellite makes one complete revolution around the earth. Because the orbit plane must pass through the earth's center of gravity (Kepler's First Law), the satellite passes through the earth's equatorial plane twice each sidereal day. The satellite is north of the equatorial plane for half of each sidereal day, and south of it for the other half.

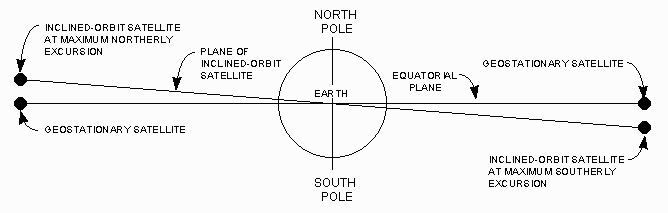From a point on the earth's surface, the satellite appears to oscillate along its north-south axis at a rate of one cycle per sidereal day.

• Secondly, for reasons beyond the scope of this article (conservation of angular momentum), the orbit assumes a slightly elliptical shape. As a result, the satellite no longer moves at constant velocity (Kepler's Second Law). From a point on the earth's surface, it appears to oscillate along its east-west axis at a rate of two cycles per sidereal day.

ECONOMICS

From the point of view of a satellite owner, the economics of this situation are compelling. On one hand, the revenue derived from leasing transponder time on an inclined-orbit satellite is considerably less than the revenue which could be realized from a truly geostationary satellite. On the other hand, propellent usage is cut dramatically, thereby extending the useful life of the satellite, often by several years. The potential revenue to be derived from this extended life more than offsets the revenue lost through reduced transponder pricing.

It comes as no surprise, then, that many satellite owners have allowed their geostationary satellites to drift into inclined orbits.

BUT IS IT GEOSTATIONARY?

        Geostationary Satellite. A geosynchronous satellite 
        whose circular and direct orbit lies in the plane of
        the earth's equator and which thus remains fixed relative
        to the earth; by extension, a satellite which remains
        approximately fixed relative to the earth. [2]

TRACKING

• EL/AZ mount. This mount is dual-axis by definition: it adjusts the antenna in the azimuth (east-west) and elevation (up-down) axes. Example:

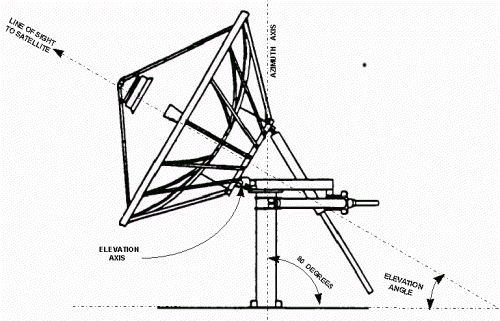Antenna with EL/AZ-Mount (Andrew Corporation)

• Polar mount. A polar mount can be used for tracking if it is equipped to allow adjustment about the declination axis throughout the full range of the satellite's figure-8 pattern. Example:

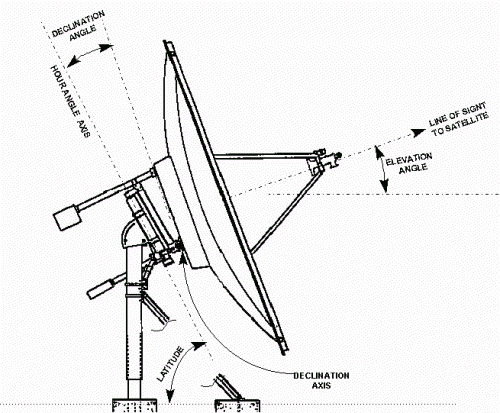Antenna with Dual-Axis Polar Mount (Comtech Antenna Systems, Inc.)

• Hybrid mount. Andrew manufactures a dual-axis mount which is neither EL/AZ nor polar. The up-down axis is elevation; the east-west axis falls between azimuth and hour angle.

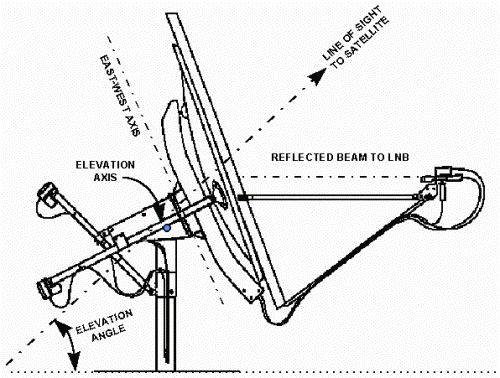Antenna with Dual-Axis Hybrid-Mount (Andrew Corporation)

-------------------------